Hp To Torque Equation
How do you calculate mechanical horsepower?To find the mechanical horsepower of a motor, use thehorsepower formula:HP = ( Torque x RPM) / 63025Torque is measured in Inch PoundsExample: The mechanical horsepower of a motor with 1000in/lbsof output torque and an output speed of 2000 RPMis:HP = (1000 x 2000) / 63025Calculated out this gives a mechanical horsepower of31.7334.(rounded to the nearest 10,000th)$100 PromotionWin $100 towards teaching supplies! We want to see your websites and blogs.Calculator PopupsCalculator IdeasWe use your calculator ideas to create new and useful online calculators.
Image credit: National Instruments CorporationThe inverse relationship between speed and means that an increase in the load (torque) on the motor will cause a decrease in speed. This can be demonstrated by the DC motor torque equation:Where:T = motor torqueV = supply voltageω = rotational speedk = motor constantR = resistanceOf course, the motor constant (k) doesn’t change, and resistance (R) in the motor windings is constant.
This article explains how torque and horsepower are related, and exposes some of. So what's the relationship between horsepower and torque when we talk.

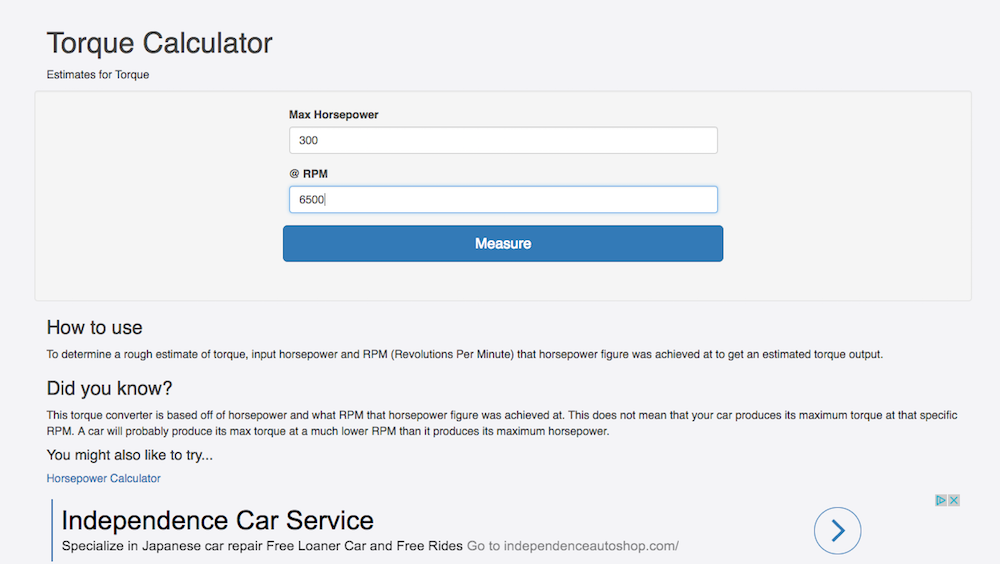
Hp To Torque Equation
Therefore, when supply voltage (V) is constant, torque (T) is inversely proportional to speed (ω).Rearranging for speed, we can see the same inverse relationship to torque:To see the derivation of the DC motor torque equation, check out.The inverse relationship means that the torque-speed curve is a descending line, with a negative slope. The torque-speed curve begins at the crossing of the Y axis, where torque is maximum and speed is zero. This is the stall torque—the maximum torque when the motor is running at nominal voltage. The curve slopes downward until it intersects the X axis—that is, zero torque and maximum speed. This point is known as the no-load speed—the speed when running at nominal voltage and zero load.Because the torque-speed curve is a straight line, it’s simple to find the torque that the motor can produce at a given speed, or conversely, to find the motor’s speed for a given load (torque) on the shaft. Recall the equation for a straight line:Where:y = value of y axis variable, to be determinedm = slope of the line; change in y divided by change in xx = value of x axis variable, givenb = y intercept; point at which the line crosses the y axisUsing this equation for the torque-speed curve, we can find the motor’s torque at a given speed. In this case, the variables in the line equation represent the following:y = torque to be determinedm = change in torque divided by change in speedx = given speedb = stall torque (value where the line crosses the y axis)The line equation can also be rearranged to find the motor’s speed at a given torque.